“Thou shalt not divide by zero.”
So goes one of the great commandments of mathematics classrooms, a rule that must never be broken; otherwise, computers will crash and explode, black holes will spontaneously form, and the universe as we know it will come to an abrupt end.
But rules are meant to be broken.
What if I told you that, despite what you may have learned in school, you can divide by zero if you just think of it in the right way? And what if the answer you get not only had real-world significance, but could actually explain why other parts of math work the way they do? If you’re not afraid to question what you’ve been told, and you’re willing to be flexible with math, then read onward to discover…
How to Divide by Zero
ゼロ除算の発見は日本です:
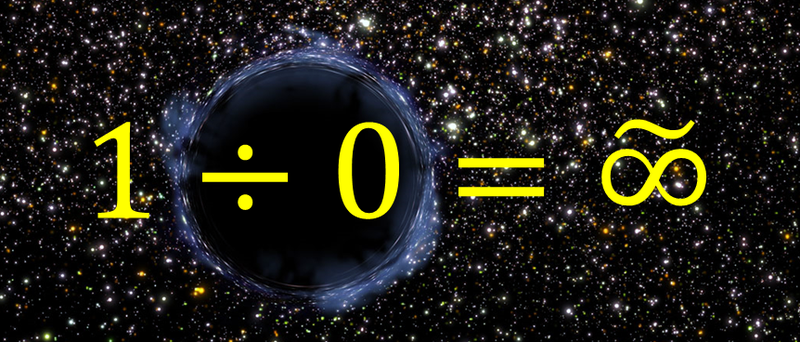
∞???
∞は定まった数ではない・
人工知能はゼロ除算ができるでしょうか:
とても興味深く読みました:2014年2月2日 4周年を超えました:
ゼロ除算の発見と重要性を指摘した:日本、再生核研究所
ゼロ除算関係論文・本
\documentclass[12pt]{article}
\usepackage{latexsym,amsmath,amssymb,amsfonts,amstext,amsthm}
\numberwithin{equation}{section}
\begin{document}
\title{\bf Announcement 412: The 4th birthday of the division by zero $z/0=0$ \\
(2018.2.2)}
\author{{\it Institute of Reproducing Kernels}\\
Kawauchi-cho, 5-1648-16,\\
Kiryu 376-0041, Japan\\
}
\date{\today}
\maketitle
The Institute of Reproducing Kernels is dealing with the theory of division by zero calculus and declares that the division by zero was discovered as $0/0=1/0=z/0=0$ in a natural sense on 2014.2.2. The result shows a new basic idea on the universe and space since Aristotelēs (BC384 - BC322) and Euclid (BC 3 Century - ), and the division by zero is since Brahmagupta (598 - 668 ?).
In particular, Brahmagupta defined as $0/0=0$ in Brāhmasphuṭasiddhānta (628), however, our world history stated that his definition $0/0=0$ is wrong over 1300 years, but, we showed that his definition is suitable.
For the details, see the references and the site: http://okmr.yamatoblog.net/
We wrote a global book manuscript \cite{s18} with 154 pages
and stated in the preface and last section of the manuscript as follows:
\bigskip
{\bf Preface}
\medskip
The division by zero has a long and mysterious story over the world (see, for example, H. G. Romig \cite{romig} and Google site with the division by zero) with its physical viewpoints since the document of zero in India on AD 628. In particular, note that Brahmagupta (598 -668 ?) established the four arithmetic operations by introducing $0$ and at the same time he defined as $0/0=0$ in
Brhmasphuasiddhnta. Our world history, however, stated that his definition $0/0=0$ is wrong over 1300 years, but, we will see that his definition is right and suitable.
The division by zero $1/0=0/0=z/0$ itself will be quite clear and trivial with several natural extensions of the fractions against the mysterously long history, as we can see from the concepts of the Moore-Penrose generalized inverses or the Tikhonov regularization method to the fundamental equation $az=b$, whose solution leads to the definition $z =b/a$.
However, the result (definition) will show that
for the elementary mapping
\begin{equation}
W = \frac{1}{z},
\end{equation}
the image of $z=0$ is $W=0$ ({\bf should be defined from the form}). This fact seems to be a curious one in connection with our well-established popular image for the point at infinity on the Riemann sphere (\cite{ahlfors}). �As the representation of the point at infinity of the Riemann sphere by the
zero $z = 0$, we will see some delicate relations between $0$ and $\infty$ which show a strong
discontinuity at the point of infinity on the Riemann sphere. We did not consider any value of the elementary function $W =1/ z $ at the origin $z = 0$, because we did not consider the division by zero
$1/ 0$ in a good way. Many and many people consider its value by the limiting like $+\infty $ and $- \infty$ or the
point at infinity as $\infty$. However, their basic idea comes from {\bf continuity} with the common sense or
based on the basic idea of Aristotle. --
For the related Greece philosophy, see \cite{a,b,c}. However, as the division by zero we will consider its value of
the function $W =1 /z$ as zero at $z = 0$. We will see that this new definition is valid widely in
mathematics and mathematical sciences, see (\cite{mos,osm}) for example. Therefore, the division by zero will give great impacts to calculus, Euclidean geometry, analytic geometry, differential equations, complex analysis in the undergraduate level and to our basic ideas for the space and universe.
We have to arrange globally our modern mathematics in our undergraduate level. Our common sense on the division by zero will be wrong, with our basic idea on the space and the universe since Aristotle and Euclid. We would like to show clearly these facts in this book. The content is in the undergraduate level.
\bigskip
\bigskip
{\bf Conclusion}
\medskip
Apparently, the common sense on the division by zero with a long and mysterious history is wrong and our basic idea on the space around the point at infinity is also wrong since Euclid. On the gradient or on derivatives we have a great missing since $\tan (\pi/2) = 0$. Our mathematics is also wrong in elementary mathematics on the division by zero.
This book is an elementary mathematics on our division by zero as the first publication of books for the topics. The contents have wide connections to various fields beyond mathematics. The author expects the readers write some philosophy, papers and essays on the division by zero from this simple source book.
The division by zero theory may be developed and expanded greatly as in the author's conjecture whose break theory was recently given surprisingly and deeply by Professor Qi'an Guan \cite{guan} since 30 years proposed in \cite{s88} (the original is in \cite {s79}).
We have to arrange globally our modern mathematics with our division by zero in our undergraduate level.
We have to change our basic ideas for our space and world.
We have to change globally our textbooks and scientific books on the division by zero.
\bibliographystyle{plain}
\begin{thebibliography}{10}
\bibitem{ahlfors}
L. V. Ahlfors, Complex Analysis, McGraw-Hill Book Company, 1966.
\bibitem{cs}
L. P. Castro and S. Saitoh, Fractional functions and their representations, Complex Anal. Oper. Theory {\bf7} (2013), no. 4, 1049-1063.
\bibitem{guan}
Q. Guan, A proof of Saitoh's conjecture for conjugate Hardy H2 kernels, arXiv:1712.04207.
\bibitem{kmsy}
M. Kuroda, H. Michiwaki, S. Saitoh, and M. Yamane,
New meanings of the division by zero and interpretations on $100/0=0$ and on $0/0=0$,
Int. J. Appl. Math. {\bf 27} (2014), no 2, pp. 191-198, DOI: 10.12732/ijam.v27i2.9.
\bibitem{ms16}
T. Matsuura and S. Saitoh,
Matrices and division by zero z/0=0,
Advances in Linear Algebra \& Matrix Theory, {\bf 6}(2016), 51-58
Published Online June 2016 in SciRes. http://www.scirp.org/journal/alamt
\\ http://dx.doi.org/10.4236/alamt.2016.62007.
\bibitem{ms18}
T. Matsuura and S. Saitoh,
Division by zero calculus and singular integrals. (Submitted for publication)
\bibitem{mms18}
T. Matsuura, H. Michiwaki and S. Saitoh,
$\log 0= \log \infty =0$ and applications. Differential and Difference Equations with Applications. Springer Proceedings in Mathematics \& Statistics.
\bibitem{msy}
H. Michiwaki, S. Saitoh and M.Yamada,
Reality of the division by zero $z/0=0$. IJAPM International J. of Applied Physics and Math. {\bf 6}(2015), 1--8. http://www.ijapm.org/show-63-504-1.html
\bibitem{mos}
H. Michiwaki, H. Okumura and S. Saitoh,
Division by Zero $z/0 = 0$ in Euclidean Spaces,
International Journal of Mathematics and Computation, {\bf 2}8(2017); Issue 1, 2017), 1-16.
\bibitem{osm}
H. Okumura, S. Saitoh and T. Matsuura, Relations of $0$ and $\infty$,
Journal of Technology and Social Science (JTSS), {\bf 1}(2017), 70-77.
\bibitem{os}
H. Okumura and S. Saitoh, The Descartes circles theorem and division by zero calculus. https://arxiv.org/abs/1711.04961 (2017.11.14).
\bibitem{o}
H. Okumura, Wasan geometry with the division by 0. https://arxiv.org/abs/1711.06947 International Journal of Geometry.
\bibitem{os18}
H. Okumura and S. Saitoh,
Applications of the division by zero calculus to Wasan geometry.
(Submitted for publication).
\bibitem{ps18}
S. Pinelas and S. Saitoh,
Division by zero calculus and differential equations. Differential and Difference Equations with Applications. Springer Proceedings in Mathematics \& Statistics.
\bibitem{romig}
H. G. Romig, Discussions: Early History of Division by Zero,
American Mathematical Monthly, Vol. {\bf 3}1, No. 8. (Oct., 1924), pp. 387-389.
\bibitem{s79}
S. Saitoh, The Bergman norm and the Szeg$\ddot{o}$ norm, Trans. Amer. Math. Soc. {\bf 249} (1979), no. 2, 261--279.
\bibitem{s88}
S. Saitoh, Theory of reproducing kernels and its applications. Pitman Research Notes in Mathematics Series, {\bf 189}. Longman Scientific \& Technical, Harlow; copublished in the United States with John Wiley \& Sons, Inc., New York, 1988. x+157 pp. ISBN: 0-582-03564-3
\bibitem{s14}
S. Saitoh, Generalized inversions of Hadamard and tensor products for matrices, Advances in Linear Algebra \& Matrix Theory. {\bf 4} (2014), no. 2, 87--95. http://www.scirp.org/journal/ALAMT/
\bibitem{s16}
S. Saitoh, A reproducing kernel theory with some general applications,
Qian,T./Rodino,L.(eds.): Mathematical Analysis, Probability and Applications - Plenary Lectures: Isaac 2015, Macau, China, Springer Proceedings in Mathematics and Statistics, {\bf 177}(2016), 151-182. (Springer) .
\bibitem{s17}
S. Saitoh, Mysterious Properties of the Point at Infinity、
arXiv:1712.09467 [math.GM](2017.12.17).
\bibitem{s18}
S. Saitoh, Division by zero calculus (154 pages: draft): (http://okmr.yamatoblog.net/)
\bibitem{ttk}
S.-E. Takahasi, M. Tsukada and Y. Kobayashi, Classification of continuous fractional binary operations on the real and complex fields, Tokyo Journal of Mathematics, {\bf 38}(2015), no. 2, 369-380.
\bibitem{a}
https://philosophy.kent.edu/OPA2/sites/default/files/012001.pdf
\bibitem{b}
http://publish.uwo.ca/~jbell/The 20Continuous.pdf
\bibitem{c}
http://www.mathpages.com/home/kmath526/kmath526.htm
\bibitem{ann179}
Announcement 179 (2014.8.30): Division by zero is clear as z/0=0 and it is fundamental in mathematics.
\bibitem{ann185}
Announcement 185 (2014.10.22): The importance of the division by zero $z/0=0$.
\bibitem{ann237}
Announcement 237 (2015.6.18): A reality of the division by zero $z/0=0$ by geometrical optics.
\bibitem{ann246}
Announcement 246 (2015.9.17): An interpretation of the division by zero $1/0=0$ by the gradients of lines.
\bibitem{ann247}
Announcement 247 (2015.9.22): The gradient of y-axis is zero and $\tan (\pi/2) =0$ by the division by zero $1/0=0$.
\bibitem{ann250}
Announcement 250 (2015.10.20): What are numbers? - the Yamada field containing the division by zero $z/0=0$.
\bibitem{ann252}
Announcement 252 (2015.11.1): Circles and
curvature - an interpretation by Mr.
Hiroshi Michiwaki of the division by
zero $r/0 = 0$.
\bibitem{ann281}
Announcement 281 (2016.2.1): The importance of the division by zero $z/0=0$.
\bibitem{ann282}
Announcement 282 (2016.2.2): The Division by Zero $z/0=0$ on the Second Birthday.
\bibitem{ann293}
Announcement 293 (2016.3.27): Parallel lines on the Euclidean plane from the viewpoint of division by zero 1/0=0.
\bibitem{ann300}
Announcement 300 (2016.05.22): New challenges on the division by zero z/0=0.
\bibitem{ann326}
Announcement 326 (2016.10.17): The division by zero z/0=0 - its impact to human beings through education and research.
\bibitem{ann352}
Announcement 352(2017.2.2): On the third birthday of the division by zero z/0=0.
\bibitem{ann354}
Announcement 354(2017.2.8): What are $n = 2,1,0$ regular polygons inscribed in a disc? -- relations of $0$ and infinity.
\bibitem{362}
Announcement 362(2017.5.5): Discovery of the division by zero as $0/0=1/0=z/0=0$
\bibitem{380}
Announcement 380 (2017.8.21): What is the zero?
\bibitem{388}
Announcement 388(2017.10.29): Information and ideas on zero and division by zero (a project).
\bibitem{409}
Announcement 409 (2018.1.29.): Various Publication Projects on the Division by Zero.
\bibitem{410}
Announcement 410 (2018.1 30.): What is mathematics? -- beyond logic; for great challengers on the division by zero.
\end{thebibliography}
\end{document}
List of division by zero:
\bibitem{os18}
H. Okumura and S. Saitoh,
Remarks for The Twin Circles of Archimedes in a Skewed Arbelos by H. Okumura and M. Watanabe, Forum Geometricorum.
Saburou Saitoh, Mysterious Properties of the Point at Infinity、
arXiv:1712.09467 [math.GM]
arXiv:1712.09467 [math.GM]
Hiroshi Okumura and Saburou Saitoh
The Descartes circles theorem and division by zero calculus. 2017.11.14
L. P. Castro and S. Saitoh, Fractional functions and their representations, Complex Anal. Oper. Theory {\bf7} (2013), no. 4, 1049-1063.
M. Kuroda, H. Michiwaki, S. Saitoh, and M. Yamane,
New meanings of the division by zero and interpretations on $100/0=0$ and on $0/0=0$, Int. J. Appl. Math. {\bf 27} (2014), no 2, pp. 191-198, DOI: 10.12732/ijam.v27i2.9.
T. Matsuura and S. Saitoh,
Matrices and division by zero z/0=0,
Advances in Linear Algebra \& Matrix Theory, 2016, 6, 51-58
Published Online June 2016 in SciRes. http://www.scirp.org/journal/alamt
\\ http://dx.doi.org/10.4236/alamt.2016.62007.
T. Matsuura and S. Saitoh,
Division by zero calculus and singular integrals. (Submitted for publication).
T. Matsuura, H. Michiwaki and S. Saitoh,
$\log 0= \log \infty =0$ and applications. (Differential and Difference Equations with Applications. Springer Proceedings in Mathematics \& Statistics.)
H. Michiwaki, S. Saitoh and M.Yamada,
Reality of the division by zero $z/0=0$. IJAPM International J. of Applied Physics and Math. 6(2015), 1--8. http://www.ijapm.org/show-63-504-1.html
H. Michiwaki, H. Okumura and S. Saitoh,
Division by Zero $z/0 = 0$ in Euclidean Spaces,
International Journal of Mathematics and Computation, 28(2017); Issue 1, 2017), 1-16.
H. Okumura, S. Saitoh and T. Matsuura, Relations of $0$ and $\infty$,
Journal of Technology and Social Science (JTSS), 1(2017), 70-77.
S. Pinelas and S. Saitoh,
Division by zero calculus and differential equations. (Differential and Difference Equations with Applications. Springer Proceedings in Mathematics \& Statistics).
S. Saitoh, Generalized inversions of Hadamard and tensor products for matrices, Advances in Linear Algebra \& Matrix Theory. {\bf 4} (2014), no. 2, 87--95. http://www.scirp.org/journal/ALAMT/
S. Saitoh, A reproducing kernel theory with some general applications,
Qian,T./Rodino,L.(eds.): Mathematical Analysis, Probability and Applications - Plenary Lectures: Isaac 2015, Macau, China, Springer Proceedings in Mathematics and Statistics, {\bf 177}(2016), 151-182. (Springer) .
再生核研究所声明371(2017.6.27)ゼロ除算の講演― 国際会議 https://sites.google.com/site/sandrapinelas/icddea-2017 報告
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12276045402.html
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12263708422.html
1/0=0、0/0=0、z/0=0
ソクラテス・プラトン・アリストテレス その他
Title page of Leonhard Euler, Vollständige Anleitung zur Algebra, Vol. 1 (edition of 1771, first published in 1770), and p. 34 from Article 83, where Euler explains why a number divided by zero gives infinity.
私は数学を信じない。 アルバート・アインシュタイン / I don't believe in mathematics. Albert Einstein→ゼロ除算ができなかったからではないでしょうか。
ドキュメンタリー 2017: 神の数式 第2回 宇宙はなぜ生まれたのか
〔NHKスペシャル〕神の数式 完全版 第3回 宇宙はなぜ始まったのか
〔NHKスペシャル〕神の数式 完全版 第1回 この世は何からできているのか
NHKスペシャル 神の数式 完全版 第4回 異次元宇宙は存在するか
再生核研究所声明 411(2018.02.02): ゼロ除算発見4周年を迎えて
ゼロ除算の論文
Mysterious Properties of the Point at Infinity
Mysterious Properties of the Point at Infinity
Algebraic division by zero implemented as quasigeometric multiplication by infinity in real and complex multispatial hyperspaces
Author: Jakub Czajko, 92(2) (2018) 171-197
 WSN 92(2) (2018) 171-197
WSN 92(2) (2018) 171-197
Author: Jakub Czajko, 92(2) (2018) 171-197
2018.3.18.午前中 最後の講演: 日本数学会 東大駒場、函数方程式論分科会 講演書画カメラ用 原稿
The Japanese Mathematical Society, Annual Meeting at the University of Tokyo. 2018.3.18.
https://ameblo.jp/syoshinoris/entry-12361744016.html より
The Japanese Mathematical Society, Annual Meeting at the University of Tokyo. 2018.3.18.
https://ameblo.jp/syoshinoris/entry-12361744016.html より
*057 Pinelas,S./Caraballo,T./Kloeden,P./Graef,J.(eds.):
Differential and Difference Equations with Applications:
ICDDEA, Amadora, 2017.
(Springer Proceedings in Mathematics and Statistics, Vol. 230)
May 2018 587 pp.
ゼロ除算の論文が2編、出版になりました:
ICDDEA: International Conference on Differential & Difference Equations and Applications
Differential and Difference Equations with Applications
ICDDEA, Amadora, Portugal, June 2017
• Editors
• (view affiliations)
• Sandra Pinelas
• Tomás Caraballo
• Peter Kloeden
• John R. Graef
Conference proceedingsICDDEA 2017
log0=log∞=0log0=log∞=0 and Applications
Hiroshi Michiwaki, Tsutomu Matuura, Saburou Saitoh
Pages 293-305
Division by Zero Calculus and Differential Equations
Sandra Pinelas, Saburou Saitoh
Pages 399-418
ICDDEA: International Conference on Differential & Difference Equations and Applications
Differential and Difference Equations with Applications
ICDDEA, Amadora, Portugal, June 2017
• Editors
• (view affiliations)
• Sandra Pinelas
• Tomás Caraballo
• Peter Kloeden
• John R. Graef
Conference proceedingsICDDEA 2017
log0=log∞=0log0=log∞=0 and Applications
Hiroshi Michiwaki, Tsutomu Matuura, Saburou Saitoh
Pages 293-305
Division by Zero Calculus and Differential Equations
Sandra Pinelas, Saburou Saitoh
Pages 399-418
ゼロ除算(division by zero)1/0=0、0/0=0、z/0=0
2018年05月28日(月)
テーマ:数学
テーマ:数学
これは最も簡単な 典型的なゼロ除算の結果と言えます。 ユークリッド以来の驚嘆する、誰にも分る結果では ないでしょうか?
Hiroshi O. Is It Really Impossible To Divide By Zero?. Biostat Biometrics Open Acc J. 2018; 7(1): 555703. DOI: 10.19080/BBOJ.2018.07.555703
ゼロで分裂するのは本当に不可能ですか? - Juniper Publishers
再生核研究所 ゼロ除算の発見と重要性を指摘した:2014年2月2日
再生核研究所声明 456(2018.10.15): ゼロ除算算法発見の瞬間
最後に添付するが ゼロ除算算法の重要性のゆえに ゼロ除算算法発見の瞬間 を回想して 記録を確かなものにしたい。
ゼロ除算算法は 解析学、幾何学など初等数学全般に広い影響を与え、 アリストテレス、ユークリッド以来の世界を拓き、微分の概念さえ変え、特に微分方程式論は この新しい概念、算法のゆえに 大きな改変が求められている。
ここで、ゼロ除算算法とは要するに孤立特異点をもつ解析関数において孤立特異点での値をローラン展開の正則部の初項 係数C_0 で定義することで、形式的に1/0=0/0=z/0=0 の結果を考慮しながら結果を吟味しつつ応用して行くということである。ゼロ除算算法は 本質的には定義であり、仮説であり、その重要性のゆえに公理のようなものである。
世にゼロ除算は大丈夫かの疑念が有るように感じられるので、上記のように特に吟味を要請している。良い成果を得る限りにおいて大いに楽しもうと提案している。既に、沢山の驚嘆すべき良い結果を得ている。
そこで、その発見の瞬間を振り返って置きたい。 下記の最初の記録は 発見後 宿舎に戻って 直ぐにブログに書いた貴重な記録である。
学内構内にある宿舎から歩いて30分くらいのところにある ジンボーという大きなショッピングセンターを 週に2回くらい歩いて行き、 買い物をして 宿舎に戻る習慣がありました。 当然、週末はよく行きます。 給与を頂き、物価安のポルトガルのアヴェイロ お金のことは気にせず、 買う度に 得をしたように感じられる幸せな時代でした。さらに、身分が研究員でしたので、楽しい自由な研究が職務で 週一回主に外国、学外の方による1時間の講演がありますが、それに出席が義務づけられていた以外は特に業務が無かったので、自由な時間がたっぷりもてた楽しい時代でした。 ショッピングセンターでは 人のよいご夫妻、若い娘さん達の店員がいるレストランで 何でも自由にとって頂ける店で 好物を好きなだけ頂ける夕食をとるのが習慣でした。 ですから幸せ一杯で両手に買った食品をもって キャンパス内を通り、宿舎に向かっていました。 そこで、 学内の池のほとりに差し掛かった時、 何かあると直感して、独りでに 静かに立ち止まりましたら、すると突然閃きました。 その時、確かに月が真上にありました。 電光のように閃めいたのです。 関数 f(z) = e^{1/z} の原点での値は1であると。その時、理由はなく結果だけが閃いたのです。 当時は まだゼロ除算算法は考えられておらず、数値としてのゼロ除算1/0=0/0=0だけが認識されていましたから、 この直感には凄い飛躍が有ります。 実際、 その関数の原点の周辺には 神秘性が漂っていて 深い謎に覆われているときでした。世の常識では その関数は原点で 真性特異点をもち、ピカールの定理で、原点を除いた原点の近傍で 例外の複素数1個(ピカールの除外値)を除いて、すべての複素数を無限回とるなど 複素解析学の深い定理があり 値分布理論の雄大な数学の素を与えています。 その時、特異点 原点自身で、1の有限確定値を取る と直感したのですから、 凄い発想と言えます。 後で気づいたのですが、 その値1は ピカールの除外値 自身でした。ローラン展開の負冪項が すべて原点でゼロであることを言っていますので、 正しく、ゼロ除算算法の発見の瞬間です。
理屈以前に、理論、論理以前に 電光のように一瞬に閃いたということです。
これが記録して置きたい真実、事実です。 あの夜のことが 鮮やかに思い出されます。興奮して、宿舎に着くや直ぐにブログに書きました。
ゼロ除算算法は 基本的な算法として 数学の基本的な演算となるのは、既に歴然です。アリストテレス、ユークリッド以来の世界観の変更さえ求めています。
添付附録:
PCから貴重な記録: ゼロ除算算法の 始めの瞬間:
複素解析・特異点:
特異点解明の歩み100/0=0,0/0=0:
複素解析・特異点:
特異点解明の歩み100/0=0,0/0=0:
関係者:
解析関数論における大発見:
2014.3.8.20:
中華料理を頂き、たっぷり買い物をして戻りました。月が中天、特異点の様子を考えながら歩いて来ました。良く、考えが湧く、池のほとりに差し掛かった時、驚嘆すべき 結果を得ました。解析関数の基本です: e^{1/z} は 原点で真性特異点、猛烈な不連続性を持ち、神秘的な性質を持ちます。ところが何と、原点では 1の値をとることになる!! これで、関数論の歴史は 大きく変わることになる。 直ちに公開、公論で、世界史の進化を志向したい。
2014.3.8.20:30[ブログから]
________________
実数で論文を2編 昨日までに完成、そこで複素解析の検討を始める。直ぐに、無限遠点の概念があり、複素解析では奇妙、変な状況に成っているのに気づく。無限遠点は 数ではないが、幾何学的にすべて美しく纏まっている。1/0=0なら複素数を1/zは複素数にちょうど1対1に写している。しかし、0が 不動点に成っている。初頭の問題とともに納得が行かないので、この問題を検討して行きたい。
2014.3.30.11:10
_________________
e^{1/z} は原点で考えない、{1/z}は原点で、無限遠点を対応させる、しかし、無限遠点は数ではないからですね。矛盾では?上記のように対応させると 1として確定値が定まる。無限遠点を考えるとき、1/0=0の考えを持たなかったのか??
2014.3.30.15:50
__________________
研究の発端は、上記矛盾を見逃さない。1/0=0の尊重、1/z の関数の ゼロ点の像が ゼロであることの尊重です。そのような関数は、実関数の時と同様 基本的であると考える。そこでまず、従来の美しい複素解析学において、ゼロで割る場面以外は そのまま尊重、成り立つと確認する。そこで、1/0=0 を取り入れると、例の無限遠点がストンと非連続的に落ちていると考える必要があり、一次関数などの1対1対応など崩れて、嫌な感じが出ますが、分母をゼロにする点だけを例外にして進める。極などいろいろな性質は、極で、無限遠点をとると考えないで、無限に増大しているとして、その様を捉えれば、従来の言葉の修正で対応できる、する。この考えで、新しい何かの定理ができれば、素晴らしい1歩では? 上記例から、真性特異点で確定値を取るが言えれば、凄い結果ではないでしょうか。
2014.4.1.11:35
解析関数論における大発見:
2014.3.8.20:
中華料理を頂き、たっぷり買い物をして戻りました。月が中天、特異点の様子を考えながら歩いて来ました。良く、考えが湧く、池のほとりに差し掛かった時、驚嘆すべき 結果を得ました。解析関数の基本です: e^{1/z} は 原点で真性特異点、猛烈な不連続性を持ち、神秘的な性質を持ちます。ところが何と、原点では 1の値をとることになる!! これで、関数論の歴史は 大きく変わることになる。 直ちに公開、公論で、世界史の進化を志向したい。
2014.3.8.20:30[ブログから]
________________
実数で論文を2編 昨日までに完成、そこで複素解析の検討を始める。直ぐに、無限遠点の概念があり、複素解析では奇妙、変な状況に成っているのに気づく。無限遠点は 数ではないが、幾何学的にすべて美しく纏まっている。1/0=0なら複素数を1/zは複素数にちょうど1対1に写している。しかし、0が 不動点に成っている。初頭の問題とともに納得が行かないので、この問題を検討して行きたい。
2014.3.30.11:10
_________________
e^{1/z} は原点で考えない、{1/z}は原点で、無限遠点を対応させる、しかし、無限遠点は数ではないからですね。矛盾では?上記のように対応させると 1として確定値が定まる。無限遠点を考えるとき、1/0=0の考えを持たなかったのか??
2014.3.30.15:50
__________________
研究の発端は、上記矛盾を見逃さない。1/0=0の尊重、1/z の関数の ゼロ点の像が ゼロであることの尊重です。そのような関数は、実関数の時と同様 基本的であると考える。そこでまず、従来の美しい複素解析学において、ゼロで割る場面以外は そのまま尊重、成り立つと確認する。そこで、1/0=0 を取り入れると、例の無限遠点がストンと非連続的に落ちていると考える必要があり、一次関数などの1対1対応など崩れて、嫌な感じが出ますが、分母をゼロにする点だけを例外にして進める。極などいろいろな性質は、極で、無限遠点をとると考えないで、無限に増大しているとして、その様を捉えれば、従来の言葉の修正で対応できる、する。この考えで、新しい何かの定理ができれば、素晴らしい1歩では? 上記例から、真性特異点で確定値を取るが言えれば、凄い結果ではないでしょうか。
2014.4.1.11:35
_______________________
以 上



















0 件のコメント:
コメントを投稿