Why students need more 'math talk'
Matthew Campbell, West Virginia University
Published
(The Conversation is an independent and nonprofit source of news, analysis and commentary from academic experts.)
Matthew Campbell, West Virginia University and Johnna Bolyard, West Virginia University
RECOMMENDED VIDEO
(THE CONVERSATION) Test scores, school report cards and Facebook posts complaining about homework problems often drive critiques of how math is taught in schools.
Amid the debates, it has become increasingly clear that one ingredient is necessary for success: opportunities for students to talk about math. Unfortunately, these are often lacking in U.S. classrooms.
We are both math education researchers. While we focus on different levels of the K-12 span, a common theme across our work is the role of talk in math classrooms – what talk can sound like, how talk impacts student learning, and how teachers can support math talk.
Want to support your student’s understanding of math? Talking will play a critical role. And a good place to start is to talk about math yourself.
Why talking matters
taught in schools.
Amid the debates, it has become increasingly clear that one ingredient is necessary for success: opportunities for students to talk about math. Unfortunately, these are often lacking in U.S. classrooms.
We are both math education researchers. While we focus on different levels of the K-12 span, a common theme across our work is the role of talk in math classrooms – what talk can sound like, how talk impacts student learning, and how teachers can support math talk.
Want to support your student’s understanding of math? Talking will play a critical role. And a good place to start is to talk about math yourself.
Why talking matters
For some educators and researchers, learning math means coming to know and use terms and procedures in order to quickly solve problems. Others may prioritize learning the range of ways to solve a given problem. Others, still, point to the value of skills to solve problems that may come up in “the real world.”
Those are all important aspects of mathematical proficiency, but we believe that learning to communicate about the subject is an equally important goal.
By “math talk,” we mean sharing, analyzing and making sense of math. Students might discuss their strategies for solving a problem, explaining not only what they did but also the reasoning behind their work. They can also make observations, pose questions and express uncertainties.
It’s also key for students listen to their peers – to understand what they did and respond with a comment or question. In the process, disagreements or errors might emerge. These are not things to avoid; rather, they are opportunities to extend learning. Engaging in math talk helps all involved understand the ideas at hand.
Research, such as the work led by education researchers Suzanne Chapin and Beth Herbel-Eisenmann, has shown how math talk supports learning. It can improve memory and understanding; aid the development of language and social skills; and boost confidence and interest in math.
Learning math is not a process of acquiring a set of facts or procedures, but a process of becoming one who participates in a community that does mathematical work. People use math to collaborate and communicate with others. They make sense of problems that are interesting and complex. They justify their ideas and work to convince others of the validity of those ideas. They make sense of the justifications posed by others to understand, critique and build on their thinking. These skills are not reserved for mathematicians or engineers, but apply to wide range of careers.
How to support math talk
The classroom in which math talk is not supported is a familiar scene: desks in rows, a teacher presenting a new procedure, and students working individually, focused on copying problems, getting an answer, and doing so as quickly as possible.
There are many ways in which a teacher can foster a classroom rich in opportunities for math talk. One recommendation, from research in cognitive science, is the use of “worked examples” – problems that have been worked out by someone else, perhaps a hypothetical student – to improve student learning. For example, students can be presented with two different but correct strategies to a problem and be asked to compare and contrast them, looking for the benefits and drawbacks of each approach. As a class, students can compare their ideas and raise new questions, all facilitated by the teacher.
But math talk is not just something that can happen in a classroom. In our positions, we each often get asked by friends and family about how to help their children in math. Our answer? Talk more about math – and preferably not just about homework assignments.
Math can be found in anything in ways that are appropriate for different ages. Say you are out shopping: How many people are in the store? How high is the ceiling? How many beach balls would it take to fill up the room? How do you know? Taking the time to engage with your student around any of those questions is math talk.
Many of these questions might not have a readily available answer, and that can be a good thing. Talking about what you would need to know or do to find an answer is just as valuable, and likely even more valuable, than time spent with flash cards and apps with math “games” that only focus on speed with procedures. Blogs and social media have become spaces to share the ways in which you can be “talking math with your kids” (#tmwyk on Twitter).
Whether in second grade or in an AP calculus classroom, mathematics achievement will continue to lag without value placed on math talk.
This article is republished from The Conversation under a Creative Commons license. Read the original article here: http://theconversation.com/why-students-need-more-math-talk-104034.
ゼロ除算の発見は日本です:
∞???
∞は定まった数ではない・・・・
人工知能はゼロ除算ができるでしょうか:
とても興味深く読みました:2014年2月2日 4周年を超えました:
ゼロ除算の発見と重要性を指摘した:日本、再生核研究所
ゼロ除算関係論文・本
行ってきました:世界を変えた書物展
まず、世の数学に対する自然な心情、- 数学はダメ、数学は嫌い、興味も関心も無い、話したくない。 これでは数学界の基本的な問題であり、数学教育の基本的な過ちを表していると言えるのではないだろうか。これでは数学自身の存在が脅かされ、文化をささえるべき数学が 基本的な役割を果たしているとは言えない。多くの素敵な人たちが、 受験勉強や教育の場で 数学に虐められたと表明している。 高校時代の素敵な女性にそのように卒業後56年を経たクラス会で表明されて この声明のきっかけを得た。 ある相当な化学の教授が 数学がどうも苦手で 化学を専攻せざるを得なかったという言葉も 永く、心に響いている。高校の担任は数学で、50年を超えてクラス会に招かれているから、人物良好、尊敬される存在である。しかし、数学教育は受験の必要性に迫られて 型にはまった教育にならざるを得なかったが、当時はまだ受験は 甘い余裕のあった時代であったが、受験数学の過熱さは凄くなっている現実があるのではないだろうか。 そのような意味では、数学嫌いな人々をどんどん多く育てている現実があるのではないかと危惧している。
ところが、このような状況に対して、数学好きな人々は 己が才能の優秀性を示す好機と見て、このような風潮を助長させているような状況が 数学者に相当に見られると考えられる。
― 数学の先生が、生徒を馬鹿にするような風潮が広く見られ、多くの生徒が永く傷つき、数学と数学の先生に恨みさえ懐いている現実は 相当に見られるのではないだろうか。また数学の歴史には 競争を争ったり、自分の才能を示したいという存念があるが、研究の面では 仕方がなく、数学者が自己中心で視野も狭く、子供っぽいとの批判も 研究上やむを得ないと弁明せざるを得ない。
日本では数学ができる、頭が良い、高学歴の変な文化を育て 数学の社会的な存在基盤を弱くして 閉じた狭いエリート社会を構成している状況が広く見られる。 数学の研究、教育が 広い社会的な存在にならず、閉じた極めて小さな存在になっていると思われる。数学界のいろいろな賞等、新聞や世の話題にもならず、それらは 我々に何の意味もないと多くの人は興味を懐かず、無関心である。 数学者自身理屈っぽく、社会性がなく、変人が多く、自己中心の視野の狭い人々の集まりと 考えられているのではないだろうか。― 研究者としてやもう得ないが 教育面では改めたい。
前向きに考えれば、まず数学教育の中心を 数学の楽しさ、数学の精神を身に着ける教育への回帰を提案したい。多くの人々が数学とは 楽しいものであるとの認識を持たせるような教育を まずは 志向すべきである。 当然、数学者は真理を求める真摯な態度で 人々から尊敬される人物と見なされるべき人物を志向すべきである。- ポルトガルでは サッカーが大衆レベルで人気が有り、それ故にサッカー選手は 尊敬の 話題の中心である。 日本では 数学の愛好者が多く、数学者は 社会の話題の中心的な存在である。 これは無理としても、そのような有りようを志向するのは 良いのではないだろうか。
受験のための数学より、文化のため、文化の基礎のための数学、芸術、楽しみのための数学の視点は 大事ではないだろうか。
マスコミやマスメデア関係者は いわゆる 文系が多く、特に上記で述べたように数学嫌い、数学者嫌いの方々が多く、数学界の情報は 社会的な広がりを見せない異常な状態ではないだろうが。 それで分かり易い、スポーツ、芸能、ドラマなどの異常な氾濫を招いていて、数理科学の情報が閉ざされているのではないだろうか。これはもちろん、狭い見解である。
数学者や数学教育についてご意見など頂ければ、美しい数学のために 活かしたい。
神の数式:
神の数式が解析関数でかけて居れば、 特異点でローラン展開して、正則部の第1項を取れば、 何時でも有限値を得るので、 形式的に無限が出ても 実は問題なく 意味を有します。
物理学者如何でしょうか。
カテゴリ:カテゴリ未分類
そこで、計算機は何時、1/0=0 ができるようになるでしょうか。 楽しみにしています。 もうできる進化した 計算機をお持ちの方は おられないですね。
これは凄い、面白い事件では? 計算機が人間を超えている 例では?
面白いことを発見しました。 計算機は 正しい答え 0/0=0
を出したのに、 この方は 間違いだと 言っている、思っているようです。
0/0=0 は 1300年も前に 算術の発見者によって与えられたにも関わらず、世界史は間違いだと とんでもないことを言ってきた。 世界史の恥。 実は a/0=0 が 何時も成り立っていた。 しかし、ここで 分数の意味を きちんと定義する必要がある。 計算機は、その意味さえ知っているようですね。 計算機、人間より賢くなっている 様が 出て居て 実に 面白い。
https://steemkr.com/utopian-io/@faisalamin/bug-zero-divide-by-zero-answers-is-zero
2018.10.11.11:23
カテゴリ:カテゴリ未分類
面白いことを発見しました。 計算機は 正しい答え 0/0=0
を出したのに、 この方は 間違いだと 言っている、思っているようです。
0/0=0 は 1300年も前に 算術の発見者によって与えられたにも関わらず、世界史は間違いだと とんでもないことを言ってきた。 実は a/0=0 が 何時も成り立っていた。しかし、ここで 分数の意味を きちんと定義する必要がある。 計算機は、その意味さえ知っているようですね。 計算機、人間より賢くなっている様が 出て居て 実に面白い。
https://steemkr.com/utopian-io/@faisalamin/bug-zero-divide-by-zero-answers-is-zero
2018.10.11.11:23
ゼロ除算、ゼロで割る問題、分からない、正しいのかなど、 良く理解できない人が 未だに 多いようです。そこで、簡潔な一般的な 解説を思い付きました。 もちろん、学会などでも述べていますが、 予断で 良く聞けないようです。まず、分数、a/b は a 割る b のことで、これは 方程式 b x=a の解のことです。ところが、 b がゼロならば、 どんな xでも 0 x =0 ですから、a がゼロでなければ、解は存在せず、 従って 100/0 など、ゼロ除算は考えられない、できないとなってしまいます。 普通の意味では ゼロ除算は 不可能であるという、世界の常識、定説です。できない、不可能であると言われれば、いろいろ考えたくなるのが、人間らしい創造の精神です。 基本方程式 b x=a が b がゼロならば解けない、解が存在しないので、困るのですが、このようなとき、従来の結果が成り立つような意味で、解が考えられないかと、数学者は良く考えて来ました。 何と、 そのような方程式は 何時でも唯一つに 一般化された意味で解をもつと考える 方法があります。 Moore-Penrose 一般化逆の考え方です。 どんな行列の 逆行列を唯一つに定める 一般的な 素晴らしい、自然な考えです。その考えだと、 b がゼロの時、解はゼロが出るので、 a/0=0 と定義するのは 当然です。 すなわち、この意味で 方程式の解を考えて 分数を考えれば、ゼロ除算は ゼロとして定まる ということです。ただ一つに定まるのですから、 この考えは 自然で、その意味を知りたいと 考えるのは、当然ではないでしょうか?初等数学全般に影響を与える ユークリッド以来の新世界が 現れてきます。
ゼロ除算の誤解は深刻:
最近、3つの事が在りました。
私の簡単な講演、相当な数学者が信じられないような誤解をして、全然理解できなく、目が回っているいるような印象を受けたこと、
相当ゼロ除算の研究をされている方が、基本を誤解されていたこと、1/0 の定義を誤解されていた。
相当な才能の持ち主が、連続性や順序に拘って、4年以上もゼロ除算の研究を避けていたこと。
これらのことは、人間如何に予断と偏見にハマった存在であるかを教えている。
まずは ゼロ除算は不可能であるの 思いが強すぎで、初めからダメ、考えない、無視の気持ちが、強い。 ゼロ除算を従来の 掛け算の逆と考えると、不可能であるが 証明されてしまうので、割り算の意味を拡張しないと、考えられない。それで、 1/0,0/0,z/0 などの意味を発見する必要がある。 それらの意味は、普通の意味ではないことの 初めの考えを飛ばして ダメ、ダメの感情が 突っ走ている。 非ユークリッド幾何学の出現や天動説が地動説に変わった世界史の事件のような 形相と言える。
最近、3つの事が在りました。
私の簡単な講演、相当な数学者が信じられないような誤解をして、全然理解できなく、目が回っているいるような印象を受けたこと、
相当ゼロ除算の研究をされている方が、基本を誤解されていたこと、1/0 の定義を誤解されていた。
相当な才能の持ち主が、連続性や順序に拘って、4年以上もゼロ除算の研究を避けていたこと。
これらのことは、人間如何に予断と偏見にハマった存在であるかを教えている。
まずは ゼロ除算は不可能であるの 思いが強すぎで、初めからダメ、考えない、無視の気持ちが、強い。 ゼロ除算を従来の 掛け算の逆と考えると、不可能であるが 証明されてしまうので、割り算の意味を拡張しないと、考えられない。それで、 1/0,0/0,z/0 などの意味を発見する必要がある。 それらの意味は、普通の意味ではないことの 初めの考えを飛ばして ダメ、ダメの感情が 突っ走ている。 非ユークリッド幾何学の出現や天動説が地動説に変わった世界史の事件のような 形相と言える。
2018.9.22.6:41
ゼロ除算の4つの誤解:
ゼロ除算の4つの誤解:
1. ゼロでは割れない、ゼロ除算は 不可能である との考え方に拘って、思考停止している。 普通、不可能であるは、考え方や意味を拡張して 可能にできないかと考えるのが 数学の伝統であるが、それができない。
2. 可能にする考え方が 紹介されても ゼロ除算の意味を誤解して、繰り返し間違えている。可能にする理論を 素直に理解しない、 強い従来の考えに縛られている。拘っている。
3. ゼロ除算を関数に適用すると 強力な不連続性を示すが、連続性のアリストテレス以来の 連続性の考えに囚われていて 強力な不連続性を受け入れられない。数学では、不連続性の概念を明確に持っているのに、不連続性の凄い現象に、ゼロ除算の場合には 理解できない。
4. 深刻な誤解は、ゼロ除算は本質的に定義であり、仮定に基づいているので 疑いの気持ちがぬぐえず、ダメ、怪しいと誤解している。数学が公理系に基づいた理論体系のように、ゼロ除算は 新しい仮定に基づいていること。 定義に基づいていることの認識が良く理解できず、誤解している。
George Gamow (1904-1968) Russian-born American nuclear physicist and cosmologist remarked that "it is well known to students of high school algebra" that division by zero is not valid; and Einstein admitted it as {\bf the biggest blunder of his life} [1]:1. Gamow, G., My World Line (Viking, New York). p 44, 1970.
再生核研究所声明 458(2018.10.29): 神の存在と信仰- 悟りへの心得
(本年、ゼロ除算についての5つの大きな講演の機会を 順調に楽しく済ませて、さらに高校時代のクラス会 ―― 我々の世代が終末に向かっていることを教えてくれた ―― に出席、ある区切りができたら、上記の構想が 独りでに纏まって来たので 表現して置きたい。この心情には触れたことが有り、ポルトガルに5年間滞在して 身に感じた心情、直観である。)
マリア様に擁かれた幼児キリスト様の像を繰り返し見てきた、出会った人々の世界観。 人々は同じような情景として 母親に擁かれた幼児を想い浮かべて欲しい。キリストも、幼児も 定かに自分がどのような存在か ぼんやりしていて分からない。 ただ大きなものに擁かれていて安心、安らかである。 人類、人間の存在も 神の存在も 同じようなものである。人類の 人間の知能などは たかが知れていて、何一つ良くは観えない 心 貧しい存在 である。 しかしながら、我々のすべてを支えておられる 大きな存在 を感じることが できる。それは信仰であり、神の存在の認識である と言える。 全てを、我々を全体として支えてくれる 大いなる存在 である。 信仰の前に おそれも、迷いもない 悟りの境地 と言える。 これがポルトガルで身に感じた宗教観である。絶対的な大きなものに対する帰依ともいえる。 確信こそ、信仰の深さと言える。
それに対して、仏教は少し違う感じを受ける。お釈迦さまは はたして 上記のような大いなるものの存在と帰依の精神を 持たれていたでしょうか。 お釈迦さまは 世の無常を解かれ、世の道理を説かれ、共感、共鳴、共存、生命の一体感を説かれ、己と環境の調和を求められ、自己と他、大いなる自然との調和を説かれたのではないでしょうか。 宗教観や世界観に一見すると大きな違いが存在して、定義にもよるが、仏教は 宗教とは言えないような側面があり、ある意味では 頼りない要素もあるように感じられる。 他方、ポルトガルの宗教では帰依することによって自己修行の道を閉ざし、 安易な思想に停滞して、一神教と独善性に陥り易い面が存在するように感じられる。- そのような問題は 神の問題で 我々は 天上のことと地上のことの2元論で 神の領域の問題には 信じ、お任せするだけでよい と いうような 言葉に表現される。
他方、日本人の多くは 大いなる自然に帰っていくので 仕方ない という心情で 終末を迎えているように見える。 寂しく去っていく姿には、無情のものが感じさせられる。心もとない。 他方、かの国では天国に帰って安心のさわやかな心情が残こることになる。 - しかしながら、究極においては同じようなものだと言えるのでは ないだろうか。 - 修行が大事であることには 変わりはない。
神の存在について触れた。そのような意味での神の存在には 確信を懐いて世の中を観ている。 ところが極めて奇妙、不思議なことが感じられる。それは数学の存在である。数学とは何かについて深く論じたが、――
No.81, May 2012(pdf 432kb)
数学は、美しく、完全で、時間にも、エルギーにも よらない 永遠の存在 である。 どうしてそのように美しい世界が存在するのか不思議で 不思議でしかたがない。 誰がそのような世界を創ったのか と問えば、神の創られたもの と考えざるを得ないのではないだろうか。 そのような意味で 数学とは神学であり、数学の研究とは 神との対話を求めていると言える。 しかしながら、実は 人間の存在 とは そのようなものであると言える。 ―― 人の生きるは 真智への愛 にある。 真智とは 神の意志のことである。
以 上
God’s most important commandment
never-divide-by-zero-meme-66
Even more important than “thou shalt not eat seafood”
Published by admin, on October 18th, 2011 at 3:47 pm. Filled under: Never Divide By Zero Tags: commandment, Funny, god, zero • Comments Off on God’s most important commandment
http://thedistractionnetwork.com/.../never-divide.../page/4/
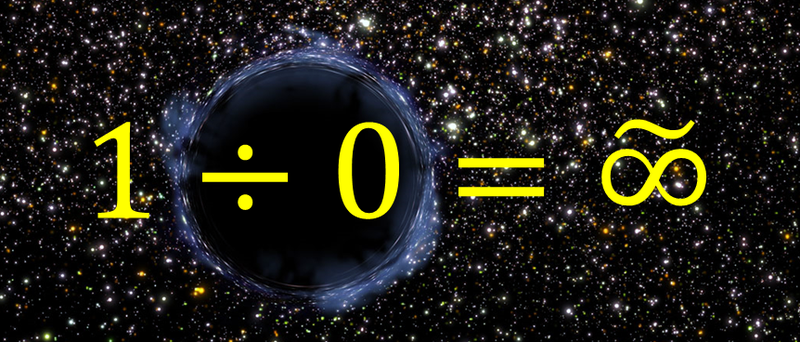
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12276045402.html
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12263708422.html
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12272721615.html
Division By Zero(ゼロ除算)1/0=0、0/0=0、z/0=0
never-divide-by-zero-meme-66
Even more important than “thou shalt not eat seafood”
Published by admin, on October 18th, 2011 at 3:47 pm. Filled under: Never Divide By Zero Tags: commandment, Funny, god, zero • Comments Off on God’s most important commandment
http://thedistractionnetwork.com/.../never-divide.../page/4/
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12276045402.html
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12263708422.html
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12272721615.html
Division By Zero(ゼロ除算)1/0=0、0/0=0、z/0=0
ゼロ除算(ゼロじょざん、division by zero)1/0=0、0/0=0、z/0=0
再生核研究所声明371(2017.6.27)ゼロ除算の講演― 国際会議 https://sites.google.com/site/sandrapinelas/icddea-2017 報告
ソクラテス・プラトン・アリストテレス その他
https://ameblo.jp/syoshinoris/entry-12328488611.html
Ten billion years ago DIVISION By ZERO:
https://www.facebook.com/notes/yoshinori-saito/ten-billion-years-ago-division-by-zero/1930645683923690/
One hundred million years ago DIVISION By ZERO
https://www.facebook.com/.../one-hundred-million-years-ago
























































0 件のコメント:
コメントを投稿