Euclidean Division of Polynomials: Theorem and Proof mes (62) in mathematics • 昨年

Euclidean Division of Polynomials: Theorem and Proof

mes (62) in mathematics •  昨年

In this video I go over further into Euclidean Division and this time look at the theorem and algorithm for univariate (i.e. single-variable) polynomials. The theorem is very similar to that for integers which I covered in my earlier video, in that both cases require proving that the associated Euclidean Division Algorithm is valid to ultimately prove that the theorem is valid. The main difference between the two algorithms is that for the integer case each step involved adding 1 to the quotient; whereas for the polynomial case we need to apply a special value, s, to ensure that the degree of the remainder keeps decreasing incrementally.

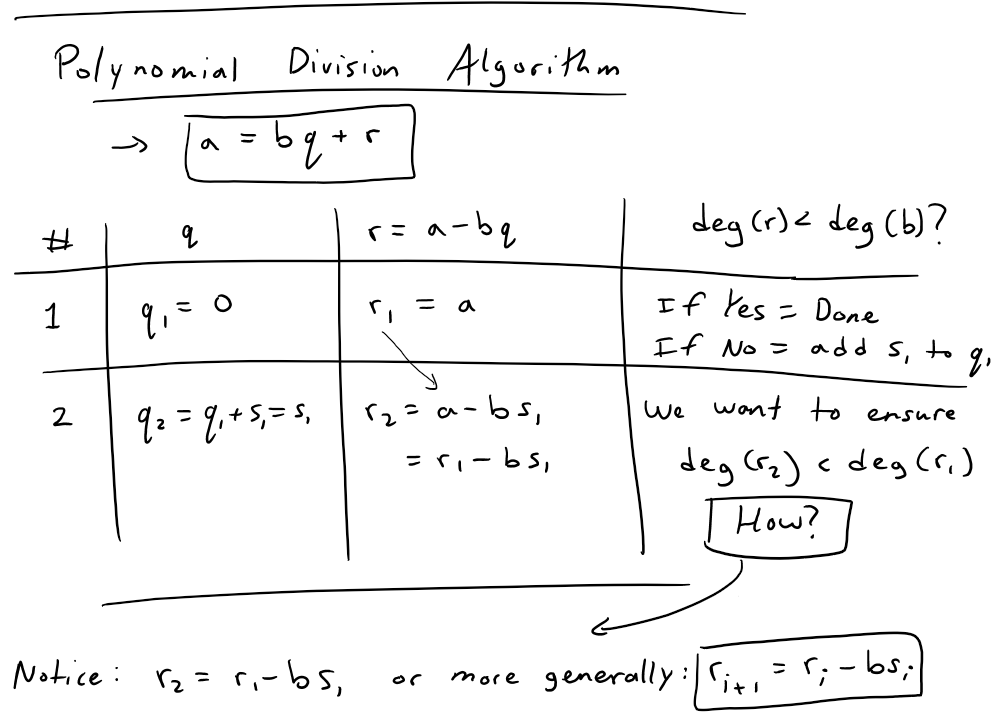
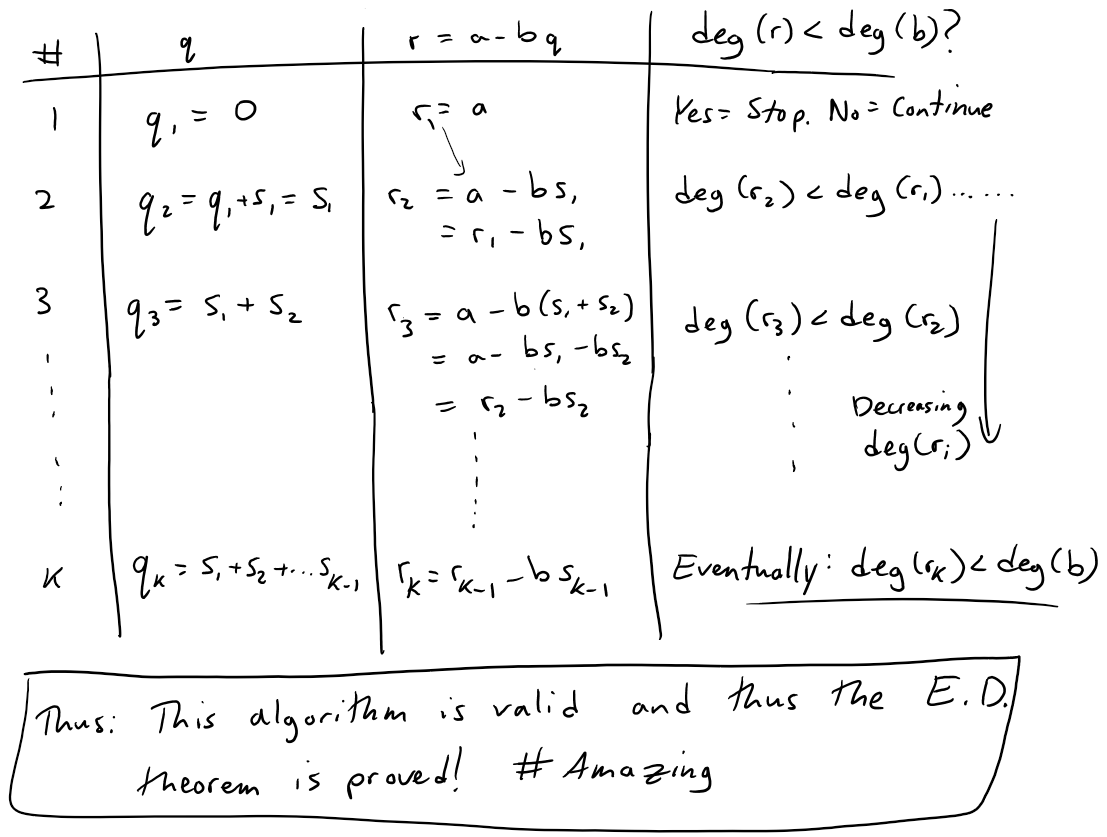
The Euclidean Theorem for Division of Polynomials is that for any two univariate polynomials a and b, where b is not equal to 0 (to avoid the case of a/0 or dividing by zero) there are associated polynomials q and r called the quotient and remainder, respectively, such that a = bq + r and the degree of r is less than the degree of b. Note also that in case the remainder is 0 and the degree of b is 0, deg(0) is defined as negative. The Division Algorithm involves starting from q = 0 and then applying the theorem formula to obtain r and checking if the degree of r is less than the degree of b. If it is, then we are done. If it is not, then we add a special polynomial s, which I show is such that involves the leading coefficients of both the remainder and the divisor, b, as well as their degrees (or number of their highest power). The selection of s is such that the remainder keeps subtracting by 1 its degree until ultimately it is less than the degree of b. Thus ultimately the algorithm is proved and thus so is the theorem!

The theorem and associated algorithm is the basis for polynomial long division where a/b = q + r/b and can be rearranged so that theorem holds true a = bq + r. This is a truly fascinating concept and make sure to walk-through both the algorithm as well as several examples to make Euclidean Division as clear as possible, so make sure to watch this video!

---

Watch on VidMe: https://vid.me/q9cAE

Watch on BitChute: https://www.bitchute.com/video/WkV0InjrXtRG/

Download PDF Notes: https://1drv.ms/b/s!As32ynv0LoaIh4EItHHqj75MMNeJbQ

---

View Video Notes Below!

---

Download These Notes: Link is in Video Description.
View These Notes as an Article: https://steemit.com/@mes
Subscribe via Email: http://mes.fm/subscribe
Donate! ☺ https://mes.fm/donate

Reuse of My Videos:

• Feel free to make use of / re-upload / monetize my videos as long as you provide a link to the original video.

Fight Back Against Censorship:

• Bookmark sites/channels/accounts and check periodically
• Remember to always archive website pages in case they get deleted/changed.

Check out my Reddit and Voat Math Forums:

• Reddit: https://reddit.com/r/AMAZINGMathStuff
• Voat: https://voat.co/v/AMAZINGMathStuff

Buy "Where Did The Towers Go?" by Dr. Judy Wood: https://mes.fm/judywoodbook
Follow My # FreeEnergy Video Series: https://mes.fm/freeenergy-playlist

USEFUL TIP: If I'm going too fast or too slow, play this video at a different speed.
§ TOP SECRET LIFE HACK: Your brain gets used to faster speed (#Try2xSpeed)

Euclidean Division For Polynomials: Theorem and Proof

Recall from my last video on Euclidean Division for Integers:

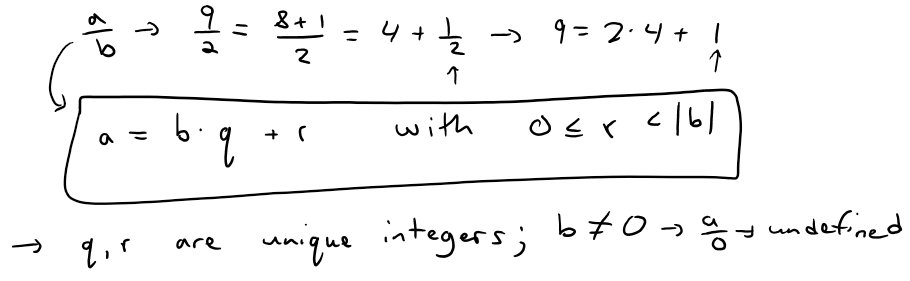
In this video I will be looking at Euclidean Division for Polynomials.

Recall that a Polynomial, more specifically a "univariate" Polynomial which consists of one variable, is of the form:

https://en.wikipedia.org/wiki/Polynomial_greatest_common_divisor#Euclidean_division

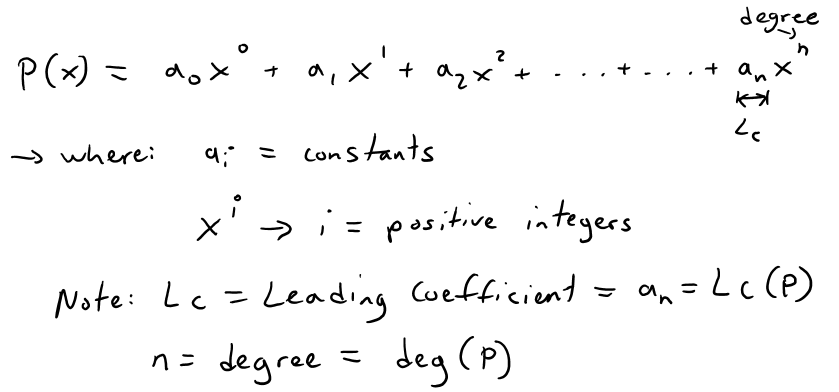
Retrieved: 20 September 2017
Archive: https://archive.is/y4IYZ

Polynomial greatest common divisor

…

Euclidean division

Euclidean division of polynomials, which is used in Euclid's algorithm for computing GCDs, is very similar to Euclidean division of integers. Its existence is based on the following theorem: Given two univariate polynomials a and b ≠ 0 defined over a field, there exist two polynomials q (the quotient) and r (the remainder) which satisfy

a = bq + r

and

deg(r) < deg(b),

where "deg(...)" denotes the degree and the degree of 0 is defined as negative.

Moreover, q and r are uniquely defined by these relations.

The difference from Euclidean division of the integers is that, for the integers, the degree is replaced by the absolute value, and that to have uniqueness one has to suppose that r is non-negative. The rings for which such a theorem exists are called Euclidean domains.

Like for the integers, the Euclidean division of the polynomials may be computed by the long division algorithm. This algorithm is usually presented for paper-and-pencil computation, but it works well on computers, when formalized as follows (note that the names of the variables correspond exactly to the regions of the paper sheet in a pencil-and-paper computation of long division). In the following computation "deg" stands for the degree of its argument (with the convention deg(0)<0), and "lc" stands for the leading coefficient, the coefficient of the highest degree of the variable.

The proof of the validity of this algorithm relies on the fact that during the whole "while" loop, we have a = bq + r and deg(r) is a non-negative integer that decreases at each iteration. Thus the proof of the validity of this algorithm also proves the validity of Euclidean division.

Now let's prove that the algorithm works, and hence prove Euclidean Division.

https://steemit.com/mathematics/@mes/euclidean-division-of-polynomials-theorem-and-proof

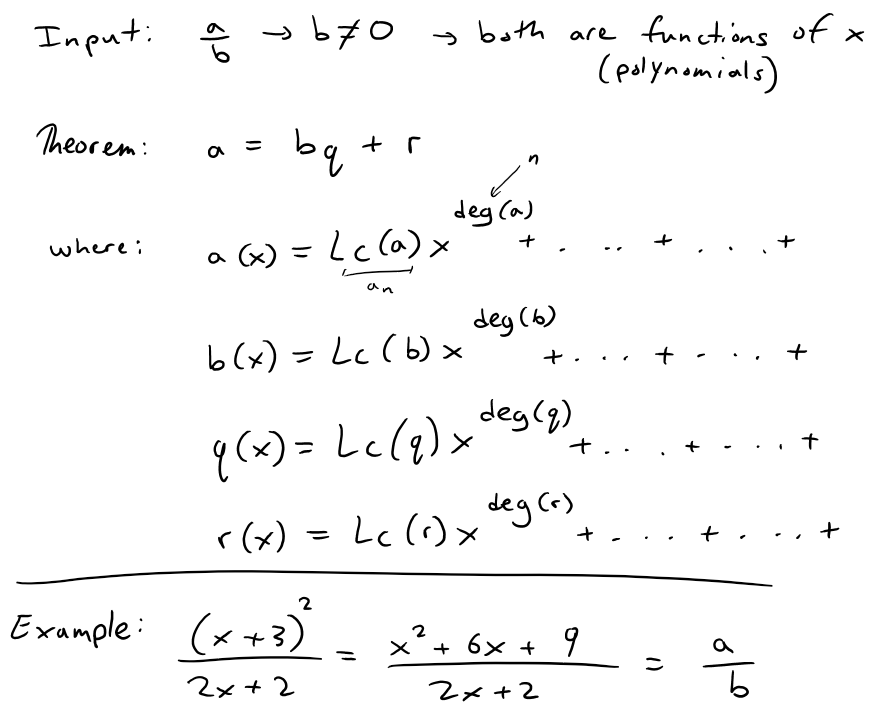
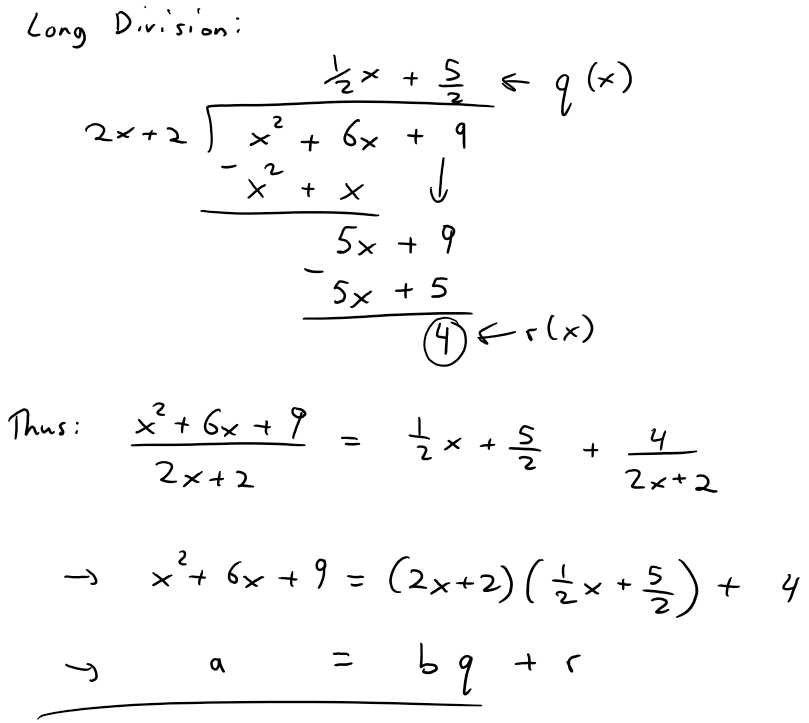
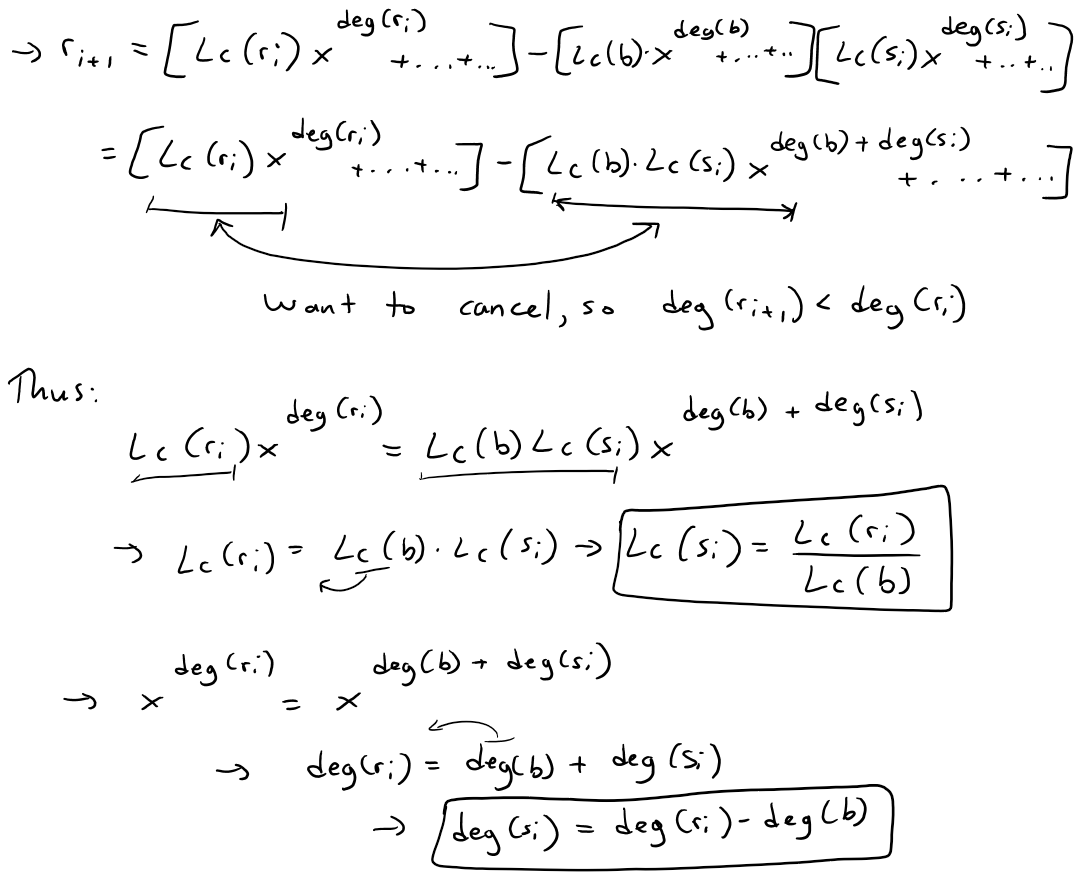
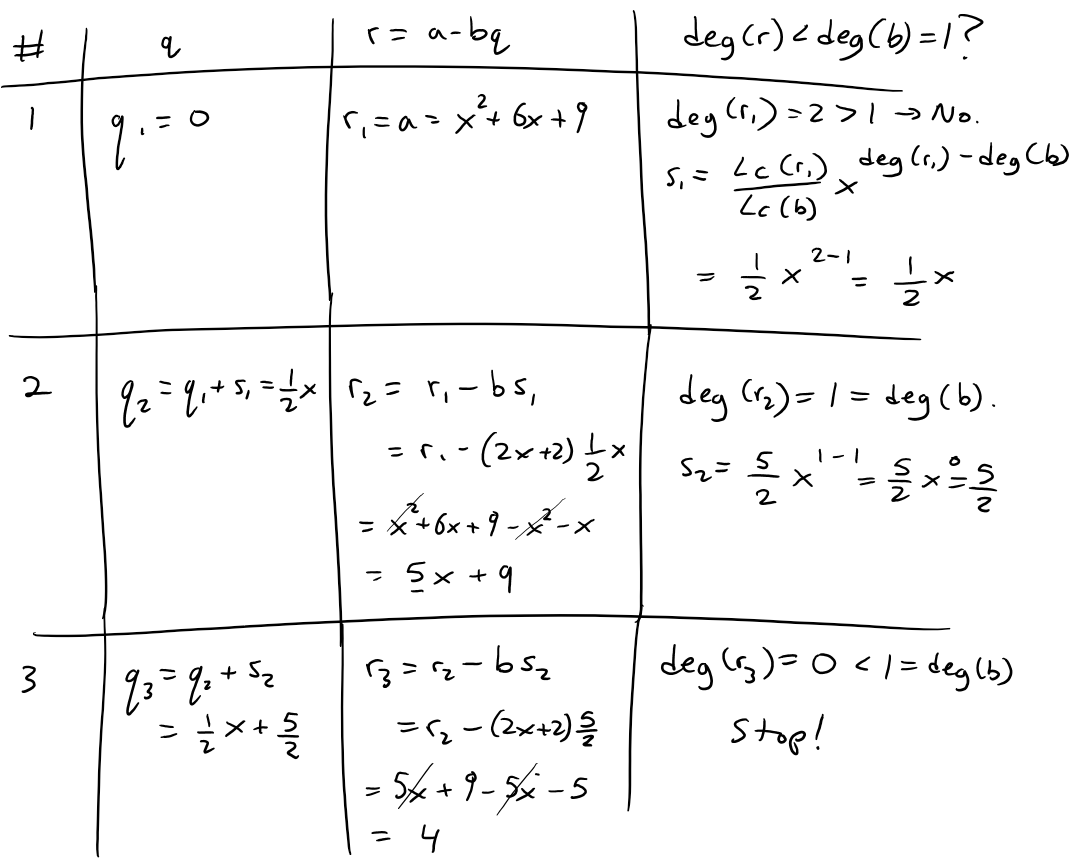
ゼロ除算の発見は日本です：

∞？？？    

∞は定まった数ではない・・・

人工知能はゼロ除算ができるでしょうか：

とても興味深く読みました：2014年2月2日　4周年を超えました：

ゼロ除算の発見と重要性を指摘した：日本、再生核研究所

ゼロ除算関係論文・本

https://ameblo.jp/syoshinoris/entry-12370797278.html

ダ・ヴィンチの名言 格言｜無こそ最も素晴らしい存在

https://systemincome.com/7521

                     

ゼロ除算の発見はどうでしょうか：
Black holes are where God divided by zero：

再生核研究所声明371（2017.6.27）ゼロ除算の講演―　国際会議　
https://ameblo.jp/syoshinoris/entry-12287338180.html

1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12276045402.html
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12263708422.html
1/0=0、0/0=0、z/0=0
http://ameblo.jp/syoshinoris/entry-12272721615.html
Division By Zero（ゼロ除算）1/0=0、0/0=0、z/0=0

https://ameblo.jp/syoshinoris/entry-12392596876.html

ゼロ除算（ゼロじょざん、division by zero）1/0=0、0/0=0、z/0=0

https://ameblo.jp/syoshinoris/entry-12394775733.html

ソクラテス・プラトン・アリストテレス　その他
https://ameblo.jp/syoshinoris/entry-12328488611.html

ドキュメンタリー 2017: 神の数式 第２回 宇宙はなぜ生まれたのか
https://www.youtube.com/watch?v=iQld9cnDli4
〔NHKスペシャル〕神の数式 完全版 第3回 宇宙はなぜ始まったのか
https://www.youtube.com/watch?v=DvyAB8yTSjs&t=3318s
〔NHKスペシャル〕神の数式 完全版 第1回 この世は何からできているのか
https://www.youtube.com/watch?v=KjvFdzhn7Dc
NHKスペシャル 神の数式 完全版 第4回 異次元宇宙は存在するか
https://www.youtube.com/watch?v=fWVv9puoTSs

再生核研究所声明 411(2018.02.02): 　ゼロ除算発見4周年を迎えて
https://ameblo.jp/syoshinoris/entry-12348847166.html

再生核研究所声明 416(2018.2.20): 　ゼロ除算をやってどういう意味が有りますか。何か意味が有りますか。何になるのですか　－　回答
再生核研究所声明 417(2018.2.23): 　ゼロ除算って何ですか　－　中学生、高校生向き　回答
再生核研究所声明 418(2018.2.24): 　割り算とは何ですか？　ゼロ除算って何ですか　－　小学生、中学生向き　回答
再生核研究所声明 420(2018.3.2): ゼロ除算は正しいですか，合っていますか、信用できますか　－　回答

2018.3.18．午前中　最後の講演：　日本数学会　東大駒場、函数方程式論分科会　講演書画カメラ用　原稿
The Japanese Mathematical Society, Annual Meeting at the University of Tokyo. 2018.3.18.
https://ameblo.jp/syoshinoris/entry-12361744016.html より

9月18日（火） 14:10～15:00

和算とゼロ除算

齋藤三郎・奥村 博

京都大学数理解析研究所 111 号室

（講演精神・要旨）

https://note.mu/ysaitoh/n/n1d38a681644f

再生核研究所声明 424(2018.3.29): 　レオナルド・ダ・ヴィンチとゼロ除算

再生核研究所声明 427(2018.5.8): 神の数式、神の意志　そしてゼロ除算

アインシュタインも解決できなかった「ゼロで割る」問題

http://matome.naver.jp/odai/2135710882669605901

Title page of Leonhard Euler, Vollständige Anleitung zur Algebra, Vol. 1 (edition of 1771, first published in 1770), and p. 34 from Article 83, where Euler explains why a number divided by zero gives infinity.

https://notevenpast.org/dividing-nothing/

私は数学を信じない。　アルバート・アインシュタイン / I don't believe in mathematics. Albert Einstein→ゼロ除算ができなかったからではないでしょうか。

1423793753.460.341866474681。                            

Einstein's Only Mistake: Division by Zero

http://refully.blogspot.jp/2012/05/einsteins-only-mistake-division-by-zero.html

ゼロ除算、ゼロで割る問題、分からない、正しいのかなど、　良く理解できない人が　未だに　多いようです。そこで、簡潔な一般的な　解説を思い付きました。　もちろん、学会などでも述べていますが、　予断で　良く聞けないようです。まず、分数、a/b は　a 　割る b　のことで、これは　方程式 b x=a　の解のことです。ところが、　b　がゼロならば、　どんな　xでも　0 x =0　ですから、a　がゼロでなければ、解は存在せず、　従って　100/0　など、ゼロ除算は考えられない、できないとなってしまいます。　普通の意味では　ゼロ除算は　不可能であるという、世界の常識、定説です。できない、不可能であると言われれば、いろいろ考えたくなるのが、人間らしい創造の精神です。　基本方程式　b x=a　が　b　がゼロならば解けない、解が存在しないので、困るのですが、このようなとき、従来の結果が成り立つような意味で、解が考えられないかと、数学者は良く考えて来ました。　何と、　そのような方程式は　何時でも唯一つに　一般化された意味で解をもつと考える　方法があります。　Moore-Penrose　一般化逆の考え方です。　どんな行列の　逆行列を唯一つに定める　一般的な　素晴らしい、自然な考えです。その考えだと、　b　がゼロの時、解はゼロが出るので、　a/0=0　と定義するのは　当然です。　すなわち、この意味で　方程式の解を考えて　分数を考えれば、ゼロ除算は　ゼロとして定まる　ということです。ただ一つに定まるのですから、　この考えは　自然で、その意味を知りたいと　考えるのは、当然ではないでしょうか？初等数学全般に影響を与える　ユークリッド以来の新世界が　現れてきます。

ゼロ除算の誤解は深刻：

最近、3つの事が在りました。

私の簡単な講演、相当な数学者が信じられないような誤解をして、全然理解できなく、目が回っているいるような印象を受けたこと、
相当ゼロ除算の研究をされている方が、基本を誤解されていたこと、1/0　の定義を誤解されていた。
相当な才能の持ち主が、連続性や順序に拘って、４年以上もゼロ除算の研究を避けていたこと。

これらのことは、人間如何に予断と偏見にハマった存在であるかを教えている。
​まずは　ゼロ除算は不可能であるの　思いが強すぎで、初めからダメ、考えない、無視の気持ちが、強い。　ゼロ除算を従来の　掛け算の逆と考えると、不可能であるが　証明されてしまうので、割り算の意味を拡張しないと、考えられない。それで、　1/0,0/0,z/0　などの意味を発見する必要がある。　それらの意味は、普通の意味ではないことの　初めの考えを飛ばして　ダメ、ダメの感情が　突っ走ている。　非ユークリッド幾何学の出現や天動説が地動説に変わった世界史の事件のような　形相と言える。

２０１８．９．２２．６：４１
ゼロ除算の４つの誤解：

１．      ゼロでは割れない、ゼロ除算は　不可能である　との考え方に拘って、思考停止している。　普通、不可能であるは、考え方や意味を拡張して　可能にできないかと考えるのが　数学の伝統であるが、それができない。

２．      可能にする考え方が　紹介されても　ゼロ除算の意味を誤解して、繰り返し間違えている。可能にする理論を　素直に理解しない、　強い従来の考えに縛られている。拘っている。

３．      ゼロ除算を関数に適用すると　強力な不連続性を示すが、連続性のアリストテレス以来の　連続性の考えに囚われていて　強力な不連続性を受け入れられない。数学では、不連続性の概念を明確に持っているのに、不連続性の凄い現象に、ゼロ除算の場合には　理解できない。

４．      深刻な誤解は、ゼロ除算は本質的に定義であり、仮定に基づいているので　疑いの気持ちがぬぐえず、ダメ、怪しいと誤解している。数学が公理系に基づいた理論体系のように、ゼロ除算は　新しい仮定に基づいていること。　定義に基づいていることの認識が良く理解できず、誤解している。

George Gamow (1904-1968) Russian-born American nuclear physicist and cosmologist remarked that "it is well known to students of high school algebra" that division by zero is not valid; and Einstein admitted it as {\bf the biggest blunder of his life} [1]：1. Gamow, G., My World Line (Viking, New York). p 44, 1970.